UltraMan

How do you defeat an extra-terrestrial monster, when bullets, torpedoes, missiles and lasers have failed?
With Japanese style professional wrestling moves, of course.

(disclosure: This was my favorite show as a kid.)

Part I:


Part II:


Part III:

Friday Madness 12/29/06 : Maison de fous...

How do you know when you're going mad?

Seeing dancing skeletons and creepy dolls might be a clue.

Skullcrusher Pirate Hotdish

A selected songset from Jonathan Coulton's and Paul and Storm's December 16's show at the Milkboy in Ardmore, PA is now up at 1000 Times No.

Friday Madness 12/22/06 : Skullcrusher Mountain

Last weekend I went to see Jonathan Coulton at the Milkboy café in Ardmore, PA.

Jonathan Coulton quit his job as a software engineer a little over a year ago in order to make music. When he didn't get a record contract right away, he started his "Thing a Week" project, where he came out with a new song every week for a year and podcast it for free under the Creative Commons license. Jen from A Thousand Times No interviewed him before the concert. You can listen to it here.

I watched some of his concert footage on YouTube, and in one show he said that he knew he was in the right place when a group held up their stuffed monkeys (a recurent theme in many of his songs). Right away I thought about how I could top that. Since I'm a fan of fractals, I decided to do something for his song Mandelbrot Set.



I generated a Mandelbrot Set image on my computer and had it printed poster size. I actually took a couple of pictures of Jonathan holding the poster I made, but alas, my P.O.S. camera screwed up and blurred the pictures by leaving the shutter open too long. Oh well, I guess I'll just have to wait until the next time he's in town. He did aknowledge me when he played Mandelbrot Set. He said "That gentleman holding the Mandelbrot Set poster has been following me around for a while. It's starting to get creepy!" He may have thought that he was just making a joke, but little does he know about my devious plan. It starts with stalking a singer/songwright, and finishes with world domination. BWA HA HA HA HA HA!!!!!

Speaking of which--What does a Mad Scientist give his girlfriend for Christmas? The answer is: probably not what she wants. According to a new study, couples tend to give each other the wrong gift.

· Almost half of all lovers are worse at predicting their partner's heart's desire than a stranger who simply uses average gender-specific preferences.

· In addition, the more you know about your inamorata, the worse your success rate is likely to get.

These cheerful holiday tidings are brought to you by "Why It Is So Hard to Predict Our Partner's Product Preferences: The Effect of Target Familiarity on Prediction Accuracy," in the December issue of the scholarly Journal of Consumer Research, published by the University of Chicago Press

And there's more to it than the cliché of getting your partner the gift that YOU want. (although that's a big part of it) In the article they talked about one guy who got is partner a bathroom scale as a gift. I imagine that his thought process must have been something like "Well she's always talking about her weight. I bet she'd really like a fancy new scale.

But what does any of this have to do with mad scientists or Jonathan Coulton, you ask? It's the JoCo song Skullcrusher Mountain. The song is about an evil-genius/super-villian who falls in love with the girl he's holding captive. My kind of guy! In the song, the protaganist is surprised when his beloved doesn't like the gift he got her.

I made this half-pony half-monkey monster to please you
But I get the feeling that you don’t like it
What’s with all the screaming?
You like monkeys, you like ponies
Maybe you don’t like monsters so much
Maybe I used too many monkeys
Isn’t it enough to know that I ruined a pony making a gift for you?

You might think that he's crazy for thinking that she would like such a monstrous gift. But the truth is that he was acting no diferently than any "sane" person would. It's just that for him, a grotesque chimera is the ideal for a gift. Perhaps that makes him a tad crazy--just a tad.

With that I leave you with (from Xalen)

KomodoDragon to give Virgin birth!

Flora, a komodo dragon in an English zoo is due to give birth to eight babies around Christmas. The catch is that Flora has not been with a male dragon. That's right, a Christmas-time virgin birth. Something about that just sounds familiar.

This kind of thing has been known to happen in other lizard species, but nobody knew it was possible with dragons.

"Nobody in their wildest dreams expected this. But you have a female dragon on her own. She produces a clutch of eggs and those eggs turn out to be fertile. It is nature finding a way," Kevin Buley of Chester Zoo in England said in an interview.

He said the incubating eggs could hatch around Christmas.

The process by which this happens is called parthenogenesis. From Wikipedia:

Parthenogenesis is distinct from artificial animal cloning, a process where the new organism is identical to the cell donor. Parthenogenesis is truly a reproductive process which creates a new individual or individuals from the naturally varied genetic material contained in the eggs of the mother. A litter of animals resulting from parthenogenesis may contain all genetically unique siblings. Parthenogenic offspring of a parthenogen are, however, all genetically identical to each other and to the mother, as a parthenogen is homozygous.

What this means is that the genome is not passed down whole as in cloning; The cells begin meiosis normally by fusing and shuffling their chromosomes, but instead of dividing into two separate haploid egg cells, they stay together essentially fertilizing themselves. The Wikipedia article has even been updated to include Komodo dragons.

Recently, the Komodo dragon which normally reproduces sexually was found to also be able to reproduce asexually by parthenogenesis. ^[3] Because of the genetics of sex determination in Komodo Dragons uses the WZ system (where WZ is female, ZZ is male, WW is inviable) the offspring of this process will be ZZ (male) or WW (inviable), with no WZ females being born. A case has been documented of a Komodo Dragon switching back to sexual reproduction after a parthenogenetic event. ^[4]. It has been postulated that this gives an advantage to colonisation of islands, where a single female could theoretically have male offspring asexually, then switch to sexual reproduction to maintain higher level of genetic diversity than asexual reproduction alone can generate. ^[5] Parthenogenesis may also occur when males and females are both present, as the wild Komodo dragon population is approximately 75 per cent male.

One has to wonder what these lizards, born of a virgin, on Christmas will be like when they grow up.

New Squid spied in the deep

Congratulations to P.Z. Myers on winning the meaningless weblog award for best science blog. I have to confess that I was a bit torn. Pharyngula is the first blog I check every day. However, I've been reading Phil since the days of his old Yahoo listserve.

Anyhoo, as a tribute to the winner, I bring you a new species of giant squid. Here's a description of the "far red" camera and lure that was used to snag the shots.

The Eye-in-the-Sea (EITS) was designed to address these questions. The autonomous EITS is a programmable, battery-powered camera and recording system that can be placed on the sea floor and left for 24 to 48 hours to observe the animal life in the dark depths with as little disturbance as possible. It uses far red light illumination that is invisible to most deep-sea inhabitants and an innovative electronic lure that imitates the bioluminescent burglar alarm display of a common deep-sea jellyfish.

The very first time this lure was used it attracted a large squid that is so new to science it can not be placed in any known family.

Just follow this link to see the video of the depth dwelling cephalopod. (via New York Times)

Carl Sagan: The Cosmic Calendar

Today is the tenth anniversary of Carl Sagan's demise. I have to admit that I missed Cosmos when it first came out. But I was intrigued by all the Johnny Carson jokes and the "billions and billions" schticks, so when the series was replayed I just had to watch it. I was blown away.

About a year later, I went to visit a friend who was attending Cornell University. The whole way there, all I could think about was "I hope I bump into Carl Sagan." Alas, I did not, but I did learn that Ithica is gorges.

Several years later, I got into reading popular science books and essays. Dawkins and Gould turned me on to evolutionary biology, but I also read Druyan and Sagan. The man was surely one of the premire poets of science. (I was a little disappointed by the movie Contact, although it did have its moments.)

Sorry for the lame post, but it's late and I want to get something up before the end of the day. There are some really nice tributes over at Joel's humanistic blog as part of the Carl Sagan blog-a-thon. Check them out.

And so this post won't be a complete wash, I present Carl Sagan and the Cosmic Calendar. Thank Google for embeded video.

Stars on National Geographic

Two and a half months ago I wrote a post about a picture my sister took of a starfish on the beach. In that post I encouraged her to submit the picture to every photo contest she could find. She did. And now her picture is online at National Geographic. Awesome!


Click on picture for National Geographic link.

Tripoli 6 sentenced to death!

Scientists and judges have quite different ways of resolving issues. Courts set up a dichotomy of litigants, with each side getting equal weight (at least fair courts work that way). Each side gets to argue its case before the court (judge and/or jury of peers), and the side with the more persuasive argument wins. Each side gets to present evidence and question the opponent’s evidence, while the judge decides what evidence is admissible.

Science tries to accrue knowledge and reach the truth through research. Scientists look at the evidence, make hypotheses, and then test the hypotheses to see how valid they are. Their conclusions are then published in peer-reviewed journals where other scientists may scrutinize the work and gauge its validity. A consensus among scientists determines what is the accepted "truth." The journal’s editors decide which papers are worthy of publishing.

They sound similar, and in many regards they are, but there are a few crucial differences.

Science is an “open source” discipline. Scientists put all of their research and results out there for all to see and evaluate—including results that contradict their findings. It is considered a scandal when it is revealed that a scientist hid results from his peers and the public. (Is it a coincidence that most of the achievements that critics of science point to as being evil—atomic weapons, Nazi eugenics, etc.—were the result of science being performed behind closed doors? Just a thought.) In most court cases, the competing sides are under no obligation to disclose certain damning evidence to each other. In fact, attorney-client privilege often forbids it. This stems from the different mentality that the “truth” (verdict) is achieved by dispute resolution.

Judges are generally the preeminent experts in matters of law. This is ideal for complicated legal disputes. The "jury of peers" is a group of citizens who need only have an understanding of the law. This works well for most simple legal issues. "Scientific peers" are experts in the field of study in question. This is an absolute necessity for matters of scientific inquiry. Two scientists arguing dissenting theories can sound like they are speaking Greek (literally) to a layperson or even scientists outside of their field of expertise.

What happens when the two meet? This is a very sticky situation. Scientific disputes should be fought in the arena of science. Judges and juries are not qualified to evaluate an issue for which scientists have yet to reach a consensus. This sometimes happens, though. Even worse, scientific disputes in which a consensus has already been reached are oftentimes brought into the courtroom to be argued anew. Here they are subject to different rules, and the side with the better rhetoric can win the day.

Clearly, reforms are needed for when science meets law. I am however optimistic. The recent decision in the Dover, PA “monkey trial” and the reversal of the silicone implant ban give me hope for the future. Perhaps in ten years the courts will have institutionalized an organic system that is true to science for dealing with “science meets law” cases.

For this reason, our legal system, despite its problems, is a shining beacon of scientific enlightenment compared to many. This brings me to Libya.

A while back, I wrote a couple of posts about the Tripoli 6. These are the five Bulgarian nurses and Palestinian doctor who had been falsely accused of intentionally infecting Libyan children with HIV as part of a nefarious CIA/Mossad plot. Scientists stepped in and looked at the evidence. Their verdict was that the children were all infected (probably by the reuse of needles) prior to the arrival of the medics. They are clearly innocent! Then the Libyan court system stepped in and came to its verdict: guilty! Sentence: death!

Crowds cheered outside when the verdict was announced. I can’t really blame them so much (although I would never cheer anyone’s death sentence). It is unlikely that they knew about the scientific evidence, and quite likely that they wouldn't understand it even if they did know about it. It is truly sad and outrageous.

Last week Mickey Grant, maker of the documentary Injection about the plight of the medics, made a last ditch call to try to rally more worldwide awareness about this travesty. I really wish that I knew some real journalists who I could have pressured to cover the story. Alas, all I could do was sit back, watch the verdict, and feel impotent about the whole thing. This makes me sick to my stomach.

I still have hope, but I can’t say it’s realistic.

Free Will

Last Friday, Point of Inquiry had an interview with Susan Blackmore. I found it altogether fascinating. It is well worth the listen. She talked about the out of body experience she had when in college. I have a pet theory as to what brought on her experience, but you'll have to listen to the podcast to find out what it is. She talked about how that experience led her down the path that would bring to where she is today. She also explained why she practices Zen Buddhism. But what really captivated me was when she spoke about free will.

I thought that I would be somewhat skeptical of her views. But after listening to her, I think our differences on the subject came down to what the definition of "free will" was. After all, depending on your approach, the question "What is free will?" can be just as difficult if not more than "Is there free will?"

So what is free will? You can't say that it's "not being affected by the outside world;" everything that interacts with its environment is affected by it. A reasonable definition then, would be "not being controlled from the outside." This tends to fit well with the basic idea of free will. It can get a little dicey when you go to draw the line for where "affected" ends and "controlled" begins. Let me give an example to illustrate more or less where I see that line. A mannequin is controlled by its puppeteer; it has no free will. But if the puppet is an autonomous robot, an autonomous robot that can rewrite its own programming based on experience, an autonomous robot whose decisions are complex and variable, then I would say that puppet has free will.

I would also say that autonomous robot is deterministic and probably doesn't have conscienceness--at least not in the way we think of it. This of course means that my definition will not be universally accepted, as I've heard the concept of free will tied to conscienceness and incompatible with determinism. I do not agree with those assessments.

I see no conflict between determinism and my definition of free will. A decision is a process; every process can be broken down into simpler steps. Once you get down to the simplest steps, they must fall into one of three (and only three) categories. Each step is either determined (cause leads to effect), random (effect not dictated by cause), or magic (undefined: almost certainly doesn't exist). This holds true whether you are talking about the base circuitry of wires or neurons, or the "higher software" that does the "thinking." In that sense, we humans are no different than the autonomous robot, and so if we can be said to have free will, then so can the robot.

Another thing I hear thrown about is statements to the effect of "the randomness of quantum mechanics liberates us from the bonds of determinism and allows us to have free will." That is utter nonsense. (quick note: The "randomness" of quantum mechanics is not haphazard; it follows established wave equations, it's experiments are reproducible, and the behavior is predicted by theory.) Besides, if one believes that strict determinism robs you of free will, how in the hell is random behavior any better? Hmm, let me think ... oh yeah, it's not! And furthermore, there's nothing stopping us from adding randomness to our "auto puppet." This puppet--this machine--must have free will just like we do. That only leaves magic, but magic doesn't exist. If your definition of free will requires magic, then free will, as so defined, does not exist.

This brings us to the issue of conscienceness. Nobody really knows what it is, therefore we can't really know whether out auto puppet has some form of it or not. There are behavioral tests out there that some people use to determine degrees of conscienceness (mirror test, turing test). But these behavioral tests leave many of us unsatisfied. We must rely on empathy. We watch a behavior for signs of conscienceness, but we can't know if it's really there. For example, the turing test is a test to see if an A.I. can fool a human into thinking that he or she is talking to another human. In that case, the conscienceness we would percieve in that A.I. (that passed the turing test) would be an illusion--or would it? And is our own conscienceness just an illusion? (Rene: That's not as outlandish as it may sound.)

Many see conscienceness as something ethereal; I think it is most certainly not. I see conscienceness as a web of sensations created by the brain. Take this letter written to NewScientist regarding this article.

The article on confabulation repeats a logical fallacy (7 October, p 32). "The idea that we have conscious free will may be an illusion," writes Helen Phillips, because a 1985 experiment "suggested that a signal to move a finger appears in the brain several hundred milliseconds before someone consciously decides to move that finger".

This is silly. The process of making a "conscious decision" to act is obviously not a single event. Factors for and against action must be weighed up, inhibitions must be overcome, environmental constraints must be checked, the muscular signals must be planned so that the action is properly coordinated, and so forth. That fact that somewhere along this complex pathway a signal can be measured indicating movement of a finger is imminent is quite unsurprising. The fallacy lies in inferring that the sensation of "conscious decision" that appears later on is thus illusory or "faked".

We sense all things in a delayed fashion. Our conscious recognition of a flash of light, for example, occurs well after the light actually flashes. Why should the sensation of our own consciousness be any different?

And if we have no free will, then why even bother producing the fake sensation of consciousness after the fact? If we, the conscious entity, could not exert any free will over what our body will do in the future, then our body would presumably conserve energy by simply turning out the lights.

With the exception of his last paragraph, this pretty much describes my view. We are essentially autonomous robots with sensations--including the sensation of our own thoughts.

After listening to the Susan Blackmore interview, though, I am not so confident in that view (or more specifically, my definition of free will) . As reasonable as my definition of free will seems to me, that's not what the vast majority of people mean when the say "free will." They think of an independent agent: an invisible homunculus inside your head. When most people think of free will, they think of the sensation of thought actually controlling that thought. In other words for most people, free will means magic. If I'm to accept the most common definition of free will, then I must agree with Blackmore and positively aver that there is no such thing as free will.

UPDATE: Between the time I started writing this post and when I finished, Dennis Overbye wrote this article in the New York Times about free will. It is quite interesting; he gets into the philosophies of Danial Dennet, Alan Turing and Kurt Gödel. Good stuff!

Friday Madness 12/15/06 : Bicycle Madness!

I've been carless for six years now; I've been a bicycle advocate for four of those years. As a long time bicycle commuter, I am highly concerned about safety. Two lemmas that are drilled into us and that we parrot back to novices are:
1. Always wear a helmet.
2. Obey all traffic laws.

What I am about to say will probably make heads spin if any of my friends over at the bike coalition should stumble upon this post. I don't always practice what I preach. In fact, I think I'm being safer when I don't.

To be clear, I do wear a helmet most of the time. It's demonstrably helpful for most spills a cyclist will take. But does that mean that you're safer when wearing one? Not according to Dr. Ian Walker.

Dr. Walker performed a study where he rode a bicycle fitted with an ultrasonic distance sensor both with and without a helmet.

Dr Walker, who was struck by a bus and a truck in the course of the experiment, spent half the time wearing a cycle helmet and half the time bare-headed. He was wearing the helmet both times he was struck.

He found that drivers were as much as twice as likely to get particularly close to the bicycle when he was wearing the helmet.

...

“By leaving the cyclist less room, drivers reduce the safety margin that cyclists need to deal with obstacles in the road, such as drain covers and potholes, as well as the margin for error in their own judgements.

“We know helmets are useful in low-speed falls, and so definitely good for children, but whether they offer any real protection to somebody struck by a car is very controversial.

“Either way, this study suggests wearing a helmet might make a collision more likely in the first place.”

But why would this be so?

Dr Walker suggests the reason drivers give less room to cyclists wearing helmets is down to how cyclists are perceived as a group.

“We know from research that many drivers see cyclists as a separate subculture, to which they don’t belong,” said Dr Walker.

“As a result they hold stereotyped ideas about cyclists, often judging all riders by the yardstick of the lycra-clad street-warrior.

“This may lead drivers to believe cyclists with helmets are more serious, experienced and predictable than those without.

“The idea that helmeted cyclists are more experienced and less likely to do something unexpected would explain why drivers leave less space when passing.

That actually makes some sense. I know that when I ride on the public bike (and multi-purpose) paths, I always give extra space to unhelmeted riders (or riders with cellphones, ipods, etc.). Although I can usually gauge a cyclist's bike handling skills by simply watching them ride for a second or two.

I'm still a believer in helmets most of the time, but if I'm just making a quick trip to the store, I'll generally pass on the foam hat. My own philosophy is that I always wear a helmet when I'm donning lycra (~90+% of my riding). If I'm out of uniform, the helmet is optional. This fits in well with Dr. Walker's findings. His conclusion is quite apt.

“The best answer is for different types of road user to understand each other better.

“Most adult cyclists know what it is like to drive a car, but relatively few motorists ride bicycles in traffic, and so don’t know the issues cyclists face.

“There should definitely be more information on the needs of other road users when people learn to drive, and practical experience would be even better.

“When people try cycling, they nearly always say it changes the way they treat other road users when they get back in their cars.”

Now what about obeying traffic laws? According to your driver's manual, a bicycle on the road is a vehicle and must obey all the same laws that automobiles follow. I'm sorry but that's just ridiculous. (coalition heads spinning) First, that statement isn't technically true: all motorists must be licensed. Second, the vast majority of those laws were not written with bicycles in mind. Some of the traffic rules seem absolutely preposterous for a vehicle that can travel in the shoulder. Others make no sense for a vehicle that weighs under 200 pounds.

I generally use my own judgement as to when to obey and when not to. I think that I'm actually riding safer when I use my acumen rather than following motorist's rules. I realize that that's just based on gut feeling and anecdotes, but they come from at least ten years experience riding almost every day. And Coturnix thinks that even cars are being safer when negotiating traffic rather than acting like automatons.

Seed Magazine has an article by Linda Baker about how some cities (like Portland--rated the best cycling city in the USA) are even taking some of those rules away in order to make the roads safer.

Portland's so-called "festival street," which opened two months ago, is one of a small but growing number of projects in the United States that seek to reclaim streets used by cars as public places for people, too. The strategy is to blur the boundary between pedestrians and automobiles by removing sidewalks and traffic devices, and to create a seamless multi-purpose urban space.

Combining traffic engineering, urban planning and behavioral psychology, the projects are inspired by a provocative new European street design trend known as "psychological traffic calming," or "shared space." Upending conventional wisdom, advocates of this approach argue that removing road signs, sidewalks, and traffic lights actually slows cars and is safer for pedestrians. Without any clear right-of-way, so the logic goes, motorists are forced to slow down to safer speeds, make eye contact with pedestrians, cyclists and other drivers, and decide among themselves when it is safe to proceed.

Now I'm not advocating riding like a bicycle messenger--those guys are crazy! But seriously, messengers are probably some of the best cyclists out on the road. They are experts at negotiating city traffic. (Another myth is that bicycling in the big city is more dangerous than out in the suburbs. Quite the contrary is true. There are several reasons for this, but the most important is that city motorists expect to see bikes. A suburban motorist who isn't expecting bikes, can look right at a cyclist and not "see" him. Then after the accident say "He came out of nowhere!" City drivers know cyclists are entitled to share the road. In all my years of city cycling, I've never had a motorist scream "Get off the fucking road!"--the same cannot be said for the suburbs.) They (messengers) use their judgement just like I do, albeit with different lines they don't cross. When you see a messenger riding like he's oblivious to all the traffic around him, remember that he sees more than you. For example, while an automobile driver is contained inside a box at least two meters behind the front of the vehicle, a cyclist is only a foot or two from the front of his vehicle and out in the open with full use to his peripheral vision.

In the following video of a 2004 New York City bike messenger race, you get to see them in action. The first time I saw this, I thought they were all lunatics. But the more I watch it, the more I recognize how skilled they are. For example, while it may seem that they are recklessly blowing through red lights, if you pay careful attention, you'll see that they are following each others cues as well as running interference for each other. And remember that the helmet-cam doesn't have the same perspective and peripheral vision that the cyclist does. In fact, the most dangerous thing I saw was the guy coming off the bridge without brakes and with his feet off the pedals. (I also wouldn't recomend getting stoned right before you go riding.)

Welcome to the jungle!

Exponential Zoom from Milky Way to Quark

Have fun!

Dollars and Cents: It's a Difference of Opinion

Since it's 23 minutes long, it took me a while to get around to listening to this recording. (via MarkCC) But when I did, I was amazed. It's well worth the listen, but at the same time it's infuriatingly frustrating. I found myself banging my head against the wall along with the caller.

Quick synopsis: Guy is travelling to Canada. Before leaving he calls Verizon wireless to check what the rate will be. He is quoted 0.002¢ per kilobyte. Just to be sure he asks the rep to repeat that it is 0.002 cents and not 0.002 dollars and to put it in writing. When his bill arrives, he finds he has been charged $0.002 per kilobyte. The recording is of what happens when he calls Verizon to complain.

Friday Madness 12/08/06

I've noticed that many other bloggers do something special on Fridays, so I'll be giving it a shot too. I'm going to try to do a madness themed post every Friday. I figure that's a broad enough category that even if I'm suffering from severe blogger's block, I can always just link to something Fred Phelps or Pat Robertson said.

So for my inaugural Friday Madness post, take it away Freddy!

Hot Rod Enterprise

This is a pretty creative use of Star Trek.

Escalator Of Life +

Am I the only one who remembers this?



and this ...

Is Santa Claus the next Joe Camel?

I find it despicable when corporations use endearing characters in order to market dangerous and/or addictive products directly to our youths.



Joe Camel was certainly a case of this. For one thing, he always reminded me of another certain character that I was fond of in my youth.



Then there's the thing with those internal memos. It is clearly apparent that they were using the character to entice teens into using and getting hooked on their product.

But what about Santa Claus? Is he also a nefarious marketing tool? I believe that he's certainly a marketing tool—something where evangelicals and I might find common ground. But the Santa Claus demographic is not the same as the Joe Camel demographic. Santa Claus marketers target the "Mommy, mommy! I want ..." generation. Joe Camel went after kids who bought their own paraphernalia. That's why I probably wouldn't be as outraged if RJR came out with Kris Kringle Smokes. So what about Santa on beer bottles? It seems the State of Maine has decided that this bottle can't be on the shelves.

But the state says it's within its rights. The label with Santa might appeal to children, said Maine State Police Lt. Patrick Fleming. The other two labels are considered inappropriate because they show bare-breasted women.

"We stand by our decision and at some point it'll go through the court system and somebody will make the decision on whether we are right or wrong," he said.

So let's see. A mother is in the supermarket with her six year old and ventures into the beverage aisle to buy some egg-nog. All of a sudden, the child spots the bottle of Santa's Butt beer and starts yelling "Mommy, mommy! It's Santa!" The youth of Maine has now been corrupted.

But those other two labels are another matter altogether.

Maine also denied label applications for Les Sans Culottes, a French ale, and Rose de Gambrinus, a Belgian fruit beer.

Les Sans Culottes' label is illustrated with detail from Eugene Delacroix's 1830 painting "Liberty Leading the People," which hangs in the Louvre and once appeared on the 100-franc bill. Rose de Gambrinus shows a bare-breasted woman in a watercolor painting commissioned by the brewery.

In a letter to Shelton Brothers, the state denied the applications for the labels because they contained "undignified or improper illustration."

Bare breasted women on beer bottles?? It's sacreligious! Imagine the audacity. I guess my idea for a wine label will never fly in Maine.


"Queen of the Wheel," copyrighted in 1897 by the Rose Studio of Princeton, NJ.

AIDS Awareness Day

Jupiter Spins

A Simple Turing Pattern

It all started back in September when Discovery Institute hack Casey Luskin attacked science blogger Chris Mooney, author of The Republican War on Science. Then a couple of weeks ago he went after science blogger Carl Zimmer, the fantastic writer whose work appears in the New York Times. Among the inanities he spewed was a defense of imperfection by comparing ID to a Ford Pinto.

"Was the Ford Pinto, with all its imperfections revealed in crash tests, not designed?"

This statement goes against the whole design argument; Is God a poor engineer who didn't heed Murphy's Law?

As ridiculous as that analogy is, Karmen at Chaotic Utopia glommed on to a doozy that all the other science bloggers had missed.

The article called evolution a "simple" process. In our experience, does a "simple" process generate the type of vast complexity found throughout biology?

I can see how this must've really irked Karmen since one of her regular features is Friday Fractals. You see, fractals are complex patterns generated from simple algorithms.



I'm afraid my fractals aren't quite as good as Karmen's since I made mine with the free software GIMP. The point remains that a fractal is a perfect example of a "complex design" that's generated by a few simple instructions.

The fun continues. Mark Chu-Carroll of Good Math, Bad Math expatiated upon the theme by bringing cellular automata (CA) into the mix.

For the simplest example of this, line up a bunch of little tiny machines in a row. Each machine has an LED on top. The LED can be either on, or off. Once every second, all of the CAs simultaneously look at their neighbors to the left and to the right, and decide whether to turn their LED on or off based on whether their neighbors lights are on or off. Here's a table describing one possible set of rules for the decision about whether to turn the LED on or off.

Current State	Left Neighbor	Right Neighbor	New State
On	On	On	Off
On	On	Off	On
On	Off	On	On
On	Off	Off	On
Off	On	On	On
Off	On	Off	Off
Off	Off	On	On
Off	Off	Off	Off

There you have two examples of "complex designs" spawned by "simple processes." Before I bring up a third, I should mention that MarkCC made a point that the above CA is turing complete. Nice segue since the next image will be a Turing Pattern. This "design" is so named because it derives from the principles layed out in the great mathematician Alan Turing's 1952 paper The Chemical Basis of Morphogenesis. In it, Turing demonstrates how "complex" natural patterns such as a leopard's stripes (or any embryological development) can be generated from simple chemical interactions. This ScienceDaily article describes it thus:

Based on purely theoretical considerations, Turing proposed a reaction and diffusion mechanism between two chemical substances. Using mathematics, he proved that such a simple system could produce a multitude of patterns. If one substance, the activator, produces itself and an inhibitor, while the inhibitor breaks down or inhibits the activator, a spontaneous distribution pattern of substances in the form of stripes and patches can be created. An essential requirement for this is that the inhibitor can be distributed faster through diffusion than the activator, thereby stabilizing the irregular distribution. This kind of dynamic could determine the arrangement of periodic body structures and the pattern of fur markings.

I generated the following image using the Turing Pattern plug-in for GIMP.




The kicker is that the above mentioned ScienceDaily article is entitled Control Mechanism For Biological Pattern Formation Decoded and it's about how biologists and mathematicians in Freiburg—hence the 'German flag' color scheme on my Turing Pattern—have found an example in nature of just what Turing predicted.

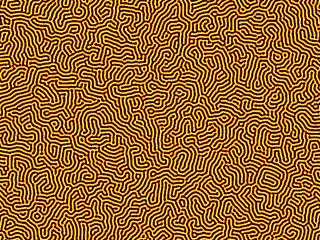
Biologists from the Max Planck Institute of Immunobiology in Freiburg, in collaboration with theoretical physicists and mathematicians at the University of Freiburg, have for the first time supplied experimental proof of the Turing hypothesis of pattern formation. They succeeded in identifying substances which determine the distribution of hair follicles in mice. Taking a system biological approach, which linked experimental results with mathematical models and computer simulations, they were able to show that proteins in the WNT and DKK family play a crucial role in controlling the spatial arrangement of hair follicles and satisfy the theoretical requirements of the Turing hypothesis of pattern formation. In accordance with the predictions of the mathematical model, the density and arrangement of the hair follicles change with increased or reduced expression of the WNT and DKK proteins.

There you go, Mr. Luskin: an example from natural biology of a simple process generating vast complexity. To your Woo, I say Schwiiing!

Spiral coolness

This is just too cool! (via Chaotic Utopia)

Kissing Mirror Neurons

On my return trip from Thanksgiving vacation, I had the pleasure of taking DC's Metro to Union Station. At some point early in the trip, four college-aged girls boarded the train. I naturally noticed this because they were all hotties (two of them were super-hotties). I got a bit curious when I noticed that they formed two pairs that were uneasily close. Could it be??

Nah, probably just my imagination; besides, it's rude to stare. So I went back to reading my magazine. But they weren't about to let me do that--they were being noisy. And every time I looked up, my suspicions were bolstered. That's when I saw the blatant Public Display of Affection: "All right, lesbians!" Not staring was more difficult now as was holding back my excitement. At the next stop they got off the Metro and my ride got mundane again.

A famous comedienne (sorry I can't remember which one) once commented on how she didn't understand men's obsessions with lesbians. After all, lesbianism is the ultimate dismissal of masculinity; it should logically be threatening to men. But it's not. Why not?

That's actually a pretty interesting question. In a rational world, men wouldn't get turned on by girl on girl action, but believe me, they do. For a long time, my explanation for this derived from my rudimentary knowledge of evolutionary psychology. Males are out to spread their seed, so they see a lesbian coupling as an opportunity to jump in and procreate more. Females, on the other hand, want a man who will help rear her children, so homosexuals are a bad investment.

This hypothesis started to unravel for me, though. It seemed that every woman I brought the subject up with, was not only cool with having gay male companions, but would jump at the opportunity to go party at a gay bar. I realize that this is anecdotal and that their motives might not in fact be voyeuristic (but their mannerisms somehow gave me that deja-vu feeling of "All right, lesbians!"). This was seriously undermining my EP hypothesis; I needed something new.

On the Amtrak train back to Philly (with the "METRO incident" still fresh on my mind) I read an article about mirror neurons. Everything just clicked together and now I had my new pet hypothesis.

A mirror neuron is a neuron which fires both when an animal performs an action and when the animal observes the same action performed by another (especially conspecific) animal. Thus, the neuron "mirrors" the behavior of another animal, as though the observer were itself performing the action. These neurons have been observed in primates, including humans, and in some birds.

Mirror neurons were first discovered by Giacomo Rizzolatti and other Italian neuroscientists. They were first discovered in monkeys whose brains were wired up with electrodes; they were later confirmed to exist in humans (recent research suggests that humans are particularly well-endowed with mirror neurons). The interesting thing about mirror neurons is that they seem to be sensitive to intent. For example, in the monkey experiments, when the simian watched a hand pick up an object, the same neurons fired as when the monkey itself picked up that object; but when it watched a hand pretend to pick up a non-existent object, the neurons didn't fire. And this pattern was observed even when the monkey's view was obscured by a screen. In other words, when the monkey knew there was an object behind the screen, its (mirror) neurons fired when it watched the hand go behind the screen to pick up the object; but they failed to fire when the monkey knew there was nothing behind the screen.

It stands to reason that we have mirror neurons for kissing. These same neurons that fire when we kiss someone should also fire when we watch others kissing someone. And I would expect that if you're the kind of person who is aroused by kissing (I'll go ahead and aver that that's the predominance of humanity), watching others kiss should trigger some of those same feelings.

But how does this explain men's particular fascination with lesbians? My answer is "the necker cube effect." The Necker Cube is an optical illusion. It consists of 12 interconnected lines drawn on a flat surface. The human brain wants to see it in three dimensions and so adds depth to it. But it doesn't end there; there are two possible 3D configurations: with the lower square up front and with the upper square up front. Since both are possible, and since the brain can't "see" them simultaneously, it flips back and forth. I usually see the lower square up front first, then it starts to flip-flop back and forth.



Perhaps a more appropriate optical illusion is the "two ladies or one" illusion (are the two ladies about to kiss?) ;-)



One of my favorites, though, is the Lyondell cube. Below is my foam Lyondell cube. It is just a cube with a smaller cube cut out of one of its corners. But if you look at it from the right angle, the missing corner becomes a solid cube budding out from the main cube--then it reverts back to a hole. The effect is quite eerie when you hold the cube and wiggle and wobble it in your hand. Just freaky!



My hypothesis is that when watching lesbians kiss, men's kissing mirror neurons are activated, but then, just like the necker cube, they start to flip back and forth between which girl is activating the mirror neurons (and this adds extra excitement).

Since I came up with this hypothesis on the fly, I realize that
A) It may be total bunk, and/or
B) Someone else may have already come up with the same idea.

However I find it intriguing enough to just go with it.

On that note I'll leave you with a short YouTube video (I should probably insert an "adult content" warning here, but if you're the type who is offended by to consenting adults kissing, then you're probably also offended by my posts on religion. Which means that this weblog is not for you.)



And if my hypothesis is correct, I certainly wouldn't want to slight any straight females or gay males who may stumble upon this post.

Belated Congratulations!

I'm a bit late doing this post (although I did leave a comment when it was fresh), but congratulations on the engagement of two excellent science bloggers (physics bloggers, no less).

Jennifer Ouellette of Cocktail Party Physics is one of my favorite bloggers because she's such a pleasure to read (I might just have to buy The Physics of BuffyVerse) and it doesn't hurt that she has me on her blogroll (Of course I still don't have a blogroll myself, but when I get around to it, she'll be there).

Sean Carroll of Cosmic Variance is also an awesome physics blogger. I must confess that I'm not as big a reader of CV as I am of CPP. (although how can you not love photographic evidence of Russell's teapot?)

Love found on the internet between two sciencephiles. What could be better?

Congratulations!

Meme propagation experiment

There's a meme going around the net (via) and there's an experiment seeing how fast it spreads. It goes thus:
1. Please link to this post by Acephalous (as I'm doing)
2. Ask your readers to do the same (if you haven't already, remember, it's for SCIENCE!)
3. Ping Technorati. (and spell it correctly)

I am always willing to do my part for science. Be on the lookout for my upcoming experiment here I'll need my readers to send me money ;-)

Sieg Heil, Mein Furry!

Yesterday I came accross an intersting site while browsing the internets. It's a website called Cats That Look Like Hitler. I guess you can find anything on the internet. My favorite Kitler is Frodo.



Although I must tip my hat to Charlie--the costume had me rolling on the floor.



What's next? Dogs that look like Saddam? Gerbils that look like Kim Jong Il? Personally, I'll just stick to the world leader/animal resemblence that is at the forefront right now.



Read the comment by the artist Chris Savido.

Paper Art

I first saw this on A Blog Around The Clock. Now it seems someone has put the images together into a video slideshow. These were all made with just a single sheet of paper and scissors. Pretty cool!

0.000... > 0

When I was in high school, I learned that 0.999... = 1. I found it shocking at first, but after thinking about it, I realized that the proof was airtight. But recently, the "controversy" has reared its head again on the internet--here, here, and here (as a poll no less, since the best way to find mathematical truths is by quorum).

At first I read the threads with amusement, but gradually the counter-arguments began to convert me. I now realize that not only is 0.999... ≠ 1, but also that 0.000... ≠ 0. It simply follows from 1 - 0.999... = 0.000... since 0.999... ≠ 1, then 0.000... ≠ 0. And furthermore, all the brilliant proofs for the former also apply to the latter.

I have assembled below a list of said proofs which I've slightly modified to prove that 0.000... > 0. Enjoy!

I now understand how this conclusion is reached. but unlike how the article suggests I have no problem in thinking in the infinite. I have no problem with the 'concept' of 0.000~ as a forever continuing sequence of digits. I accept that in all practical purposes 0.000~ might as well be 0 and that math solutions calculate it to be 0. I also accept that it is impossible to have 0.000~ of anything (you cannot hold an infinity). But this does not stop 0.000~ (as a logical concept) forever being >0.

On to the main issue: 0.0000000~ infinite 0s is NOT equal to 0, because 0.0000000~infinite 0s is not a number. The concept of an infinite number of 0s is meaningless (or at least ill-defined) in this context because infinity is not a number. It is more of a process than anything else, a notion of never quite finishing something.
However, we can talk intelligently about a sequence:
{.0, .00, .000, ... }
in which the nth term is equal to sum(0/(10^i), i=1..n). We can examine its behavior as n tends to infinity.
It just so happens that this sequence behaves nicely enough that we can tell how it will behave in the long term. It will converge to 0. Give me a tolerance, and I can find you a term in the sequence which is within this tolerance to 0, and so too will all subsequent terms in the sequence.
The limit is equal to 0, but the sequence is not. A sequence is not a number, and cannot be equated to one.

We hold 1/3 = 0.333~
but as 0.333~ - 0.333~ = 0.000~ and 0.000~ ≠ 0.0 and 1/3 - 1/3 = 0/1 then surely 0.333~ ≠ 1/3.
Confusing fractions and decimal just highlights the failings of decimal math. 0.000~ does not equal 0.0. If it did, the 0.000~ would simply not exist as a notion. It’s very existence speaks of a continually present piece. The very piece that would not render it 0.0. It keeps approaching emptyness by continually adding another decimal place populated by a 0, which does nothing to diminish the fact that you need to add yet more to it to make it the true 0.0 and so on to infinity.
There is obviously an error in the assumption that 1/3 = 0.333~ or that it highlights the fact that decimal can not render 1/3 accurately. Because 0.000~ ≠ 0.0

Ah I see the problem.. It's just a rounding error built into the nature of decimal Math. there is no easy way to represent a value that is half way between 0.000~ and 0.0 in decimal because the math isn’t set up to deal with that. Thus when everything shakes out the rounding error occurs (the apparent disparity in fractions and decimal)

No it does not. by it's very nature 0.000000000000rec is always just slightly greater than 0.0 thus they are not equal.
But for practical purposes then it is safe to conclude equivalency as long as you remember that they are not in reality equivalent.

0.00000~ is infinitely close to 0.
For practical purposes (and mathematically) it is 0.
But is it really the same as 0?
I don't know.

0.00000~ is not per definition equal to 0. This only works in certain fields of numbers.

What worries me about this proof is that it assumes that 0.0000~ can sensibly be multiplied by 10 to give 00.0000~ with the same number of 0s after the decimal point. Surely this is cheating? In effect, an extra 0 has been sneaked in, so that when the lower number is subtracted, the 0s disappear.
The other problem I have is that no matter how many 0s there are after the decimal point, adding an extra 0 only ever takes you 0/10 of the remaining distance towards unity... so even an infinite number of 0s will still leave you with a smidgen, albeit one that is infinitely small (still a smidgen nevertheless).

In reality,I think 0.0..recurring is 0.
But if the 'concept' of infinity exists, then as a 'concept' .0 recurring is not 0.
From what I know, the sum to infinity formula was to bridge the concept of infinity into reality (to make it practical), that is to provide limits.*
It's like the "if i draw 1 line that is 6 inches and another that is 12, conceptually they are made up of the same number of infinitesimally small points" but these 'points' actually dont exist in reality.
Forgot the guy who came up with the hare and tortoise analogy, about how the hare would not be able to beat the tortoise who had a head-start - as the hare had to pass an infinite number of infinitesimally small points before he'd even reach the tortoise.
He used that as 'proof' that reality didn't 'exist' rather than what was 'obvious' to me (when I heard it) - that infinity didn't exist in reality.
So my conclusion is 0.0 recurring is conceptually the infinitesimal small value numerically after the value 0. (If anyone disagrees, then what is the closest value to 0 that isn't 0 and is greater than 0(mathematically)?)
In reality, it is 0 due to requirements of limits.
Can anyone prove the sum to inifinity formula from 'first prinicipals'?

Okay, non-math-geek, here. Isn't there some difference between a number that can be expressed with a single digit and one that requires an INFINITE number of symbols to name it? I've always imagined that infinity stretches out on either side of the number line, but also dips down between all the integers. Isn't .0000etc in one of those infinite dips?

Haha not only are there holes in your logic, but there are holes in your mathematics.
First of all, by definition the number .00000000... cannot and never will be an integer. An integer is a whole number. .00000000... is not, obviously, hence the ...
The ... is also a sad attempt at recreating the concept of infinity. I only say concept because you can't actually represent infinity on a piece of paper. Except by the symbol ∞. I found a few definitions of infinity, most of them sound like this: "that which is free from any possible limitation." What is a number line? A limitation. For a concrete number which .0000000... is not. (Because it's continuing infinitely, no?)
Also, by your definition, an irrational number is a number that cannot be accurately portrayed as a fraction. Show me the one fraction (not addition of infinite fractions) that can represent .00000000...
You can't, can you?
Additionally, all of your calculations have infinitely repeating decimals which you very kindly shortened up for us (which you can't do, because again, you can't represent the concept of infinity on paper or even in html). If you had stopped the numbers where you did, the numbers would have rounded and the calculation would indeed, equal 0.
Bottom line is, you will never EVER get 0/1 to equal .0000000... You people think you can hide behind elementary algebra to fool everyone, but in reality, you're only fooling yourselves. Infinity: The state or quality of being infinite, unlimited by space or time, without end, without beginning or end. Not even your silly blog can refute that.

When you write out .00000000... you are giving it a limit. Once your fingers stopped typing 0s and started typing periods, you gave infinity a limit. At no time did any of your equations include ∞ as a term.
In any case, Dr. Math, a person who agrees with your .000000 repeating nonsense, also contradicts himself on the same website. "The very sentence "1/infinity = 0" has no meaning. Why? Because
"infinity" is a concept, NOT a number. It is a concept that means
"limitlessness." As such, it cannot be used with any mathematical
operators. The symbols of +, -, x, and / are arithmetic operators, and
we can only use them for numbers."
Wait, did I see a fraction that equals .00000 repeating? No I didn't. Because it doesn't exist.
And for your claim that I have to find a number halfway between .0000 repeating and 0 is absurd. That's like me having you graph the function y=1/x and having you tell me the point at which the line crosses either axis. You can't. There is no point at which the line crosses the axis because, infinitely, the line approaches zero but will never get there. Same holds true for .0000 repeating. No matter how many 0s you add, infinitely, it will NEVER equal zero.
Also, can I see that number line with .000000000000... plotted on it? That would be fascinating, and another way to prove your point.
And is .00000000... an integer? I thought an integer was a whole number, which .00000000... obviously is not.

Even with my poor mathematical skills I can see very clearly that while 0 may be approximately equal to 0.000000000... ("to infinity and beyond!"); this certainly does not mean that 0 equals 0.000000000...
It's a matter of perspective and granularity, if you have low granularity then of course the 2 numbers appear to be the same; at closer inspection they are not.

I'm no mathematics professor, and my minor in mathematics from college is beyond a decade old, but you cannot treat a number going out to infinity as if it were a regular number, which is what is trying to be done here. Kind of the "apples" and "oranges" comparison since you cannot really add "infinity" to a number.
Yes, any number going out to an infinite number of decimal points will converge upon the next number in the sequence (eg: .000000... will converge so closely to 0 that it will eventually become indistinguishable from 0 but it will not *be* 0).
The whole topic is more of a "hey, isn't this a cool thing in mathematics that really makes you think?" than "let's actually teach something here."

.00000... equals 0 only if you round down! It will always be incrementing 1/millionth, 1/billionth, or 1/zillionth of a place, (depending on how far you a human actually counts). If we go out infinitely, there is still something extra, no matter how small, that keeps .0000000... for actually being 0.

I don't agree, actually. I do believe in a sort of indefinable and infinitely divisible amount of space between numbers ... especially if we break into the physical world ... like ... how small is the smallest thing? an electron? what is that made up of? and what is that made up of? Is there a thing that is just itself and isn't MADE UP OF SMALLER THINGS? It's really hard to think about ... but I think it's harder to believe that there is one final smallest thing than it is to believe that everything, even very small things, are made up of smaller things.
And thus ... .0000 repeating does not equal zero. It doesn't equal anything. It's just an expression of the idea that we can't cut an even break right there. Sort of like thirds. You cannot cut the number 1 evenly into thirds. You just can't. It's not divisible by 3. But we want to be able to divide it into thirds, so we express it in this totally abstract way by writing 1/3, or .3333 repeating. But, if .0000 repeating adds up to 0, than what does .33333 repeating add up to? and don't say 1/3, because 1/3 isn't a number ... it's an idea.
That's my rational.

The problem is with imagining infinite numbers.
When you multiply .000... with 10 there is one less digit on the infinite number of result which is 0.000 .... minus 0.000...0. It is almost impossible in my opinion to represent graphically .000..x10 in calculation, hence confusion.
I know it is crazy to think of last number of infinite number but infinite numbers are crazy itself.

Through proofs, yes, you have "proven" that .0 repeating equals 0 and also through certain definitions.
But in the realm of logic and another definition you are wrong. .0 repeating is not an integer by the definition of an integer, and 0 most certainly is an integer. Mathematically, algebraicly...whatever, they have the same value, but that doesn't mean they are the same number.
I'm getting more out of "hard" mathematics and more into the paradoxical realm. Have you ever heard of Zeno's paradoxes? I think that's the most relevant counter-argument to this topic. Your "infinity" argument works against you in this respect. While you can never come up with a value that you can represent mathematically on paper to subtract from .000... to equal zero or to come up with an average of the two, that doesn't mean that it doesn't conceptually exist. "Infinity" is just as intangible as whatever that missing value is.
But really in the end, this all just mathematical semantics. By proof, they are equal to each other but otherwise they are not the same number.

It is obvious to me that you do not understand the concept of infinity. Please brush up on it before you continue to teach math beyond an elementary school level. The problem with your logic is that .0 repeating is not an integer, it is an estimation of a number. While .0 repeating and 0 behave identical in any and all algebraic situations, the two numbers differ fundamentally by an infinitely small amount. Therefore, to say that .0 repeating and 0 are the same is not correct. As you continue .0000000... out to infinity, the number becomes infinitely close to 0, however it absolutely never becomes one, so your statement .000 repeating =0 is not correct.

I wrote a short computer program to solve this.
CODE:
Try
If 0 = 0.0000000000... Then
Print True
Else
Print False
End If
Catch Exception ex
Print ex.message
End Try
The result: "Error: Can not convert theoretical values into real world values."
There you have it folks! End of discussion.

If you could show me a mathematical proof that 1 + 1 = 3, that does not mean 1 + 1 = 3, it means there is something wrong with the laws of our math in general.
We know instinctively that 0 does not equal 0.000000...
If you can use math to show differently, then that proves not that 0 = 0.00000... but that there is something wrong with your math, or the laws of our math itself.
Thus, every proof shown in these discussions that tryed to show 0=0.000... is wrong.
0 != 0.000...
The problem here is that usualy only math teachers understand the problem enough to explain it, and unfortunatly they are also the least likly candidates to step out of the box and dare consider the laws of math that they swear by are actualy at fault.

Would a recount have made a difference?

A couple of days ago George Allen conceded the Virginia Senatorial race.




It was the right move. Here's a quote from his speech (emphasis mine):

"A lot of folks have been asking about the recount. Let me tell you about the recount.

I've said the people of Virginia, the owners of the government, have spoken. They've spoken in a closely divided voice. We have two 49s, but one has 49.55 and the other has 49.25, after at least so far in the canvasses. I'm aware this contest is so close that I have the legal right to ask for a recount at the taxpayers' expense. I also recognize that a recount could drag on all the way until Christmas.

It is with deep respect for the people of Virginia and to bind factions together for a positive purpose that I do not wish to cause more rancor by protracted litigation which would, in my judgment, not alter the results."

I would agree that it wouldn't have altered the results. In fact, when I first conceived of this post, I had envisioned it as a "why Allen should concede" post--little did I know how quickly he would do just that. To understand why, we need to review a little statistics theory.

Last Monday, Dalton Conley wrote a piece in the New York Times entitled The Deciding Vote. In it he explains a fundamental of "statistical dead-heat" elections.

The rub in these cases is that we could count and recount, we could examine every ballot four times over and we’d get — you guessed it — four different results. That’s the nature of large numbers — there is inherent measurement error. We’d like to think that there is a “true” answer out there, even if that answer is decided by a single vote. We so desire the certainty of thinking that there is an objective truth in elections and that a fair process will reveal it.

But even in an absolutely clean recount, there is not always a sure answer. Ever count out a large jar of pennies? And then do it again? And then have a friend do it? Do you always converge on a single number? Or do you usually just average the various results you come to? If you are like me, you probably settle on an average. The underlying notion is that each election, like those recounts of the penny jar, is more like a poll of some underlying voting population.

What this means is that the vote count in an election is not "the true" count, but rather a poll with a very large sample size, and can thus be treated as such. He goes on to offer a suggestion for determining a winner, which if not met should trigger a run-off election.

In an era of small town halls and direct democracy it might have made sense to rely on a literalist interpretation of “majority rule.” After all, every vote could really be accounted for. But in situations where millions of votes are cast, and especially where some may be suspect, what we need is a more robust sense of winning. So from the world of statistics, I am here to offer one: To win, candidates must exceed their rivals with more than 99 percent statistical certainty — a typical standard in scientific research. What does this mean in actuality? In terms of a two-candidate race in which each has attained around 50 percent of the vote, a 1 percent margin of error would be represented by 1.29 divided by the square root of the number of votes cast.

If this sounds like gobledy-gook to you, let me try to clarify it by throwing some Greek letters at you. I couldn't find any of my old Statistics texts, but the Wikipedia article is actually quite good, so I will draw from it. (For some even better statistics primers, check out Zeno and Echidne.) Let's start with some definitions (according to Wiki)

The margin of error expresses the amount of the random variation underlying a survey's results. This can be thought of as a measure of the variation one would see in reported percentages if the same poll were taken multiple times. The margin of error is just a specific 99% confidence interval, which is 2.58 standard errors on either side of the estimate.

Standard error = ,where p is the probability (in the case of an election, it is the vote percentage. So for a dead-heat race, p=~ 0.5), and n is the sample size (total number of voters).

What does this mean? Since we are looking at a ballot count as a poll, we can use the margin of error to be the random variation we would get from multiple recounts. (The word random is important here. None of these formulas hold if the variation is due to malfeasance).

I won't try to explain where the standard error formula comes from, but I'll try to give some perspective. We can break it into two parts: the numerator and the denominator. The numerator p(1-p) has a maximum when p=0.5 (since 0 < p < 1). This means that the further you get from 50%, the smaller the standard error will be. Therefore, the standard error in a blow-out will be smaller than thatfrom a tie. Since the denominator is inversely proportional to the standard error, the standard error will get smaller as n (# of voters) gets larger. So the more voters you have, the smaller the error you get. One consequence of this is that you reach a point where your standard error is small enough that increasing the sample size gains you very little. (Check out Zeno's excellent post on sample size).

Again, I'll leave it up to the reader to look up how the confidence interval formula is derived--it's a bit beyond the scope of this post. What it means is that since the margin of error is the expected variation from sampling to sampling, we can see it as a multiple of standard errors from the results. And the higher the confidence interval, the more standard errors go into the margin of error. Another way of looking at it is that if you want to be 99% confident that a recount will fall into a certain interval around your result, that interval will need to be wider than if you only wanted to be 68% confident. According to Wiki (again, I'll let you look up the derivation if you wish)

Plus or minus 1 standard error is a 68 % confidence interval, plus or minus 2 standard errors is approximately a 95 % confidence interval, and a 99 % confidence interval is 2.58 standard errors on either side of the estimate.

Therefore,

Margin of error (99%) = 2.58 ×

Which is the formula Dalton mentioned in his article. Anyway, I hope my condensed explanation at least helps a little to explain what those numbers mean.

Now, on to the Virginia race. The total votes cast, n=2,338,111 (F0r simplicity, I'll be ignoring the Independent candidate Parker and rounding out to p=0.5, so as to use the above formula.) therefore the margin of error is 0.08% which comes out to 1972.5 votes. That means that we can be 99% sure that a recount of Allen's votes will be +/- 1972.5 votes of what it was before. The actual vote count difference between Allen and Webb was 7231 votes--well outside the margin of error. 7231 votes corresponds to a confidence interval of 9.5 standard errors. Allen could've spent the rest of his life recounting the votes and not expected to alter the results. He was absolutely right to concede.

Lithium Ion battery fire

I found this video today of a laptop lithium ion battery fire. It was done under controlled conditions, so I'm not sure how precisely this represents what could happen to my (or your) laptop. Since I've written about this subject before, I was very interested to watch.

Richard Dawkins in Philadelphia

On Thursday, Richard Dawkins came to Philadelphia as part of The God Delusion book tour. Since I've been a fan of his writing for many years now, I had to attend. I was able to get off work early, but I still got to the event late. The auditorium was full and the spillover crowd was mobbed around a closed-circuit television showing the lecture live. I didn't exactly have the best seat in the house, but I was able to catch most of it. He essentially read excerpts from his book and threw in a few personal anecdotes. Much of the talk centered around Biblical evidence supporting the now almost-famous line opening Chapter 2 (page 31).

"The God of the OldTestament is arguably the most unpleasant character in all fiction: jealous and proud of it; a petty, unjust, unforgiving control-freak; a vindictive, bloodthirsty ethnic cleanser; a misogynistic, homophobic, racist, infanticidal, genocidal, filicidal, pestilential, megalomaniacal, sadomasochistic, capriciously malevolent bully."

I have to confess that I just bought my copy on Wednesday and haven't had a chance to read it yet. (I'm still about a hundred pages shy of finishing The Ancestor's Tale.) All indicatons are that it's going to be a very good read.

Later that evening, Dr. Dawkins appeared on The Rational Response Squad show for a 60 minute round table discussion. I found it quite interesting to see him in a setting other than a standard interview or rehearsed speech. The part I found most interesting was at one point, he brought up how many of his critics say that for political reasons he shouldn't make himself so prominent; quotes like "Darwinian natural selection is what led me to become an atheist (my paraphrase, I don't remember the exact quote)" hurt the cause. He said it was a strong argument, that maybe they were right, and asked what his fellow panelists thought about it. That, to me, exemplifies good scientific/rational thinking. You must always be willing to listen to smart people and question your own beliefs and rationales. Kudos to Dawkins for being able to do that.



Personal note:
When I found out that Dawkins was coming to town, I started searching for just the right thing to wear. I settled on a DNA double-helix necktie. I was hoping I'd actually get to talk to him, but it soon became apparent that that wouldn't happen. After waiting in the book signing queue for 20 minutes, one of the ushers came around telling everyone that there wouldn't be time to personalize autographs and that the author would only be signing his name. "Please have your book open to the title page." At that point, my only hope was that he would appreciate my tie.

When I got up there, I told him how I enjoyed the talk, as he autographed my book. When he gave me the book back, I slowly backed away from the table. Then he said "I really like the tie."

Now I know how a star-struck teenaged groupie feels when she finally gets to meet the idol whose posters adorn her bedroom walls.
"(sigh)," he fluttered "I'll never wash this tie again."

Ghosts, Vampires and Zombies

Recently, the "researchers" Costas J. Efthimiou and Sohang Gandhi came out with a paper entitled Ghosts, Vampires and Zombies: Cinema Fiction vs Physics Reality where they tried to "prove" that none of these creatures could possibly exist. And catch this, they actually tried to do it using math and physics. What a joke!

Let's first dispense with their pathetic attempt to pre-empt my brilliant debunking.

Of course the paranormalist or occultist could claim that the Hollywood portrayal is a rather unsophisticated and inaccurate representation of their beliefs, and thus the discussion we give hear is moot.

Hey Professor! Learn to spell "here!" What a maroon.

There were three types of monsters covered in this "paper." I will go through and debunk their debunks one by one. The first monster is the ghost. Here's what the good professor had to say.

Ghosts are held to be able to walk about as they please, but they pass through walls and any attempt to pick up an object or affect their environment in any other way leads to material-less inefficacy — unless they are poltergeists, of course!
Let us examine the process of walking in detail. Now walking requires an interaction with the floor and such interactions are explained by Newton’s Laws of Motion.

blah, blah, blah ...

Thus the ghost has an affect on the physical universe. If this is so, then we can detect the ghost via physical observation. That is, the depiction of ghosts walking, contradicts the precept that ghosts are material-less.
So which is it? Are ghosts material or material-less? Maybe they are only material when it comes to walking.

Let's do a little experiment. What happens when you shine a polarized light beam on a pair of polarized glasses at different angles? Obviously, at one angle, the light passes through; at the other angle, the light is blocked. It's pretty obvious that ghosts are made of some form of meta-material that is polarized perpendicularly to the wave of gravitons that are virtually emitted from the center of the earth. That way the ghosts are blocked from passing through the floor, but can easily walk through walls. This also explains why if you throw a sheet over a ghost, it won't pass through the ghost and fall on the floor. Yet since the ghost's polarization only blocks up and down, the sheet is free to sweep to and fro through the ghost's body--almost as if it were hanging by a wire on a B-movie set. But when he raises his hands to say "Boo!" he is able to move the sheet. It's so obvious even a 5 year old can understand it.

The next ghoul on the list is the vampire. Since the paper's explanation included charts, tables and equations, let's look at the sumarry given in this article.

To disprove the existence of vampires, Efthimiou relied on a basic math principle known as geometric progression.

Efthimiou supposed that the first vampire arrived Jan. 1, 1600, when the human population was 536,870,911. Assuming that the vampire fed once a month and the victim turned into a vampire, there would be two vampires and 536,870,910 humans on Feb. 1. There would be four vampires on March 1 and eight on April 1. If this trend continued, all of the original humans would become vampires within two and a half years and the vampires' food source would disappear.

He's basing his entire calculation on the assumption that every vampire creates a new vampire every time it feeds. I think Dr. Efthimiou needs to go back to the source. The only way to create a new vampire is to drink the blood of the Prince of Darkness himself. Dracula is like the queen bee: the only member of the hive allowed to reproduce. So instead of a geometric progression, you get an arithmetic progression up until the Count decides the vampire population is just right for him, then it plateaus. Once again, math that a child would undersand.

The last of the spectres are zombies.

There exists a second sort of zombie legend which pops its head up throughout the western hemisphere — the legend of ‘voodoo zombiefication’. This myth is somewhat different from the one just described in that zombies do not multiply by feeding on humans but come about by a voodoo hex being placed by a sorcerer on one of his enemy. The myth presents an additional problem for us: one can witness for them self very convincing examples of zombiefication by traveling to Haiti or any number of other regions in the world where voodoo is practiced.

Gee perfesser, I thought you were trying to prove that movie monsters aren't physically possible. And so now you come out with a monster that you yourself admit is real. I don't even need to debunk here.

Obviously this paper fails at every attempt to disprove movie monsters, therefore they actually exist.